(Solved): 2. A linear transformation defined by a diagonal matrix whose diagonal entrie ...

???????

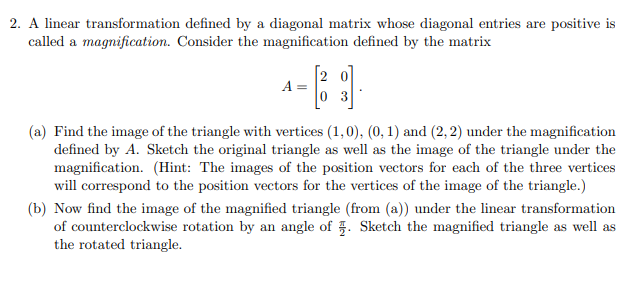
2. A linear transformation defined by a diagonal matrix whose diagonal entries are positive is called a magnification. Consider the magnification defined by the matrix \[ A=\left[\begin{array}{ll} 2 & 0 \\ 0 & 3 \end{array}\right] . \] (a) Find the image of the triangle with vertices \( (1,0),(0,1) \) and \( (2,2) \) under the magnification defined by \( A \). Sketch the original triangle as well as the image of the triangle under the magnification. (Hint: The images of the position vectors for each of the three vertices will correspond to the position vectors for the vertices of the image of the triangle.) (b) Now find the image of the magnified triangle (from (a)) under the linear transformation of counterclockwise rotation by an angle of \( \frac{\pi}{2} \). Sketch the magnified triangle as well as the rotated triangle.