(Solved): 8-28. A linear time-invariant system is described by the differential equation dt3d3y(t)+3dt2d2y ...

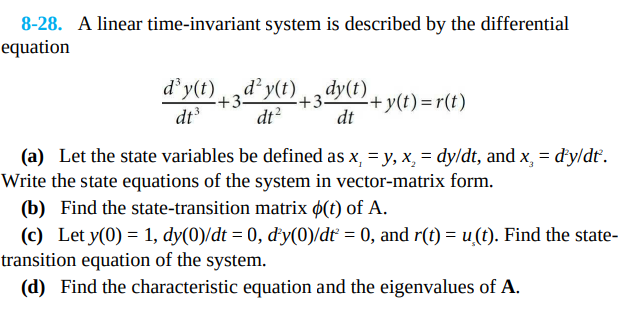
8-28. A linear time-invariant system is described by the differential equation $\frac{d^{3}y(t)}{d{t}^3}+3\frac{d^{2}y(t)}{d{t}^2}+3\frac{dy(t)}{dt}+y(t)=r(t)$ (a) Let the state variables be defined as $x_1=y,x_2=dy/dt$, and $x_3=d^{2}y/d{t}^2$. Write the state equations of the system in vector-matrix form. (b) Find the state-transition matrix $?(t)$ of $\mathrm{A}$. (c) Let $y(0)=1,dy(0)/dt=0,d^{2}y(0)/d{t}^2=0$, and $r(t)=u_s(t)$. Find the statetransition equation of the system. (d) Find the characteristic equation and the eigenvalues of $\mathbf{A}$.