(Solved): A 4-yr 6% coupon bond has a face value of 1,000,000, current market value of 1,041,420.09, dollar du ...

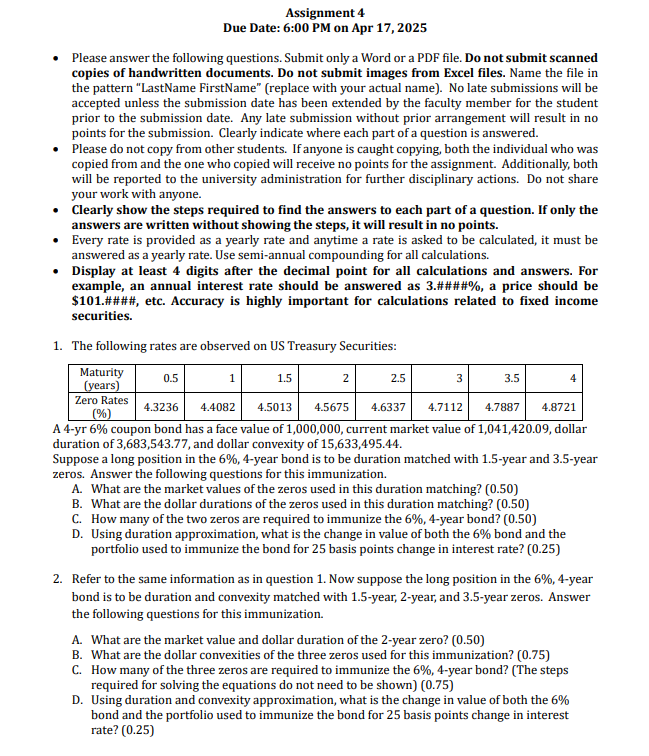
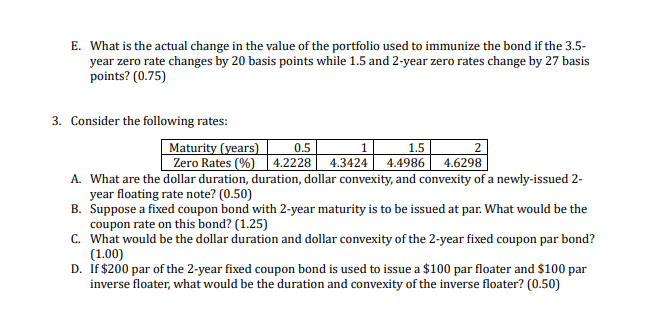
A 4-yr 6% coupon bond has a face value of 1,000,000, current market value of 1,041,420.09, dollar duration of 3,683,543.77, and dollar convexity of 15,633,495.44. Suppose a long position in the 6%, 4-year bond is to be duration matched with 1.5-year and 3.5-year zeros. Answer the following questions for this immunization. A. What are the market values of the zeros used in this duration matching? (0.50) B. What are the dollar durations of the zeros used in this duration matching? (0.50) C. How many of the two zeros are required to immunize the 6%, 4-year bond? (0.50) D. Using duration approximation, what is the change in value of both the 6% bond and the portfolio used to immunize the bond for 25 basis points change interest rate? E. What is the actual change in the value of the portfolio used to immunize the bond if the 3.5 year zero rate changes by 20 basis points while 1.5 and 2-year zero rates change by 27 basis points? (0.75) 3. Consider the following rates: A. What are the dollar duration, duration, dollar convexity, and convexity of a newly-issued 2year floating rate note? (0.50) B. Suppose a fixed coupon bond with 2-year maturity is to be issued at par. What would be the coupon rate on this bond? (1.25) C. What would be the dollar duration and dollar convexity of the 2-year fixed coupon par bond? (1.00) D. If \( \$ 200 \) par of the 2-year fixed coupon bond is used to issue a \( \$ 100 \) par floater and \( \$ 100 \) par inverse floater, what would be the duration and convexity of the inverse floater? \( (0.50) \)