Home /
Expert Answers /
Advanced Physics /
a-circular-membrane-of-radius-a-1-is-excited-at-time-t-0-with-an-initial-displacement-psi-rho-pa661
(Solved): A circular membrane of radius a=1 is excited at time t=0 with an initial displacement. \psi (\rho ,\ ...
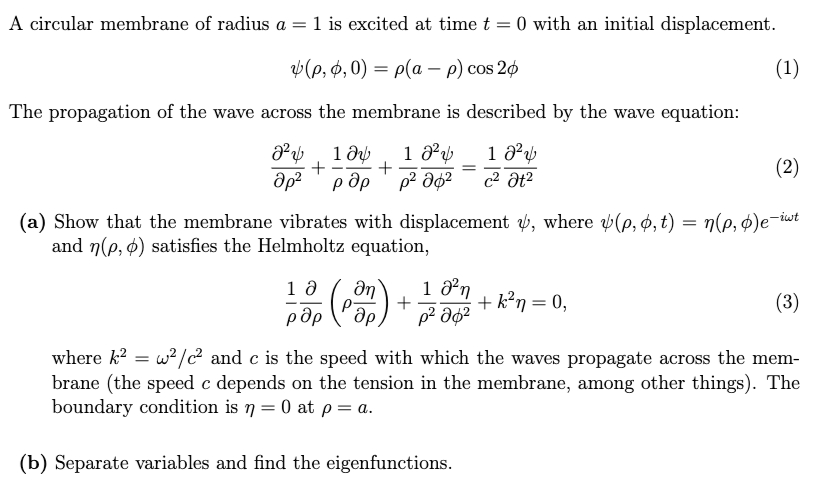
A circular membrane of radius a=1 is excited at time t=0 with an initial displacement.
\psi (\rho ,\phi ,0)=\rho (a-\rho )cos2\phi
The propagation of the wave across the membrane is described by the wave equation:
(del^(2)\psi )/(del\rho ^(2))+(1)/(\rho )(del\psi )/(del\rho )+(1)/(\rho ^(2))(del^(2)\psi )/(del\phi ^(2))=(1)/(c^(2))(del^(2)\psi )/(delt^(2))
(a) Show that the membrane vibrates with displacement \psi , where \psi (\rho ,\phi ,t)=\eta (\rho ,\phi )e^(-i\omega t)
and \eta (\rho ,\phi ) satisfies the Helmholtz equation,
(1)/(\rho )(del)/(del\rho )(\rho (del\eta )/(del\rho ))+(1)/(\rho ^(2))(del^(2)\eta )/(del\phi ^(2))+k^(2)\eta =0
where k^(2)=(\omega ^(2))/(c^(2)) and cc depends on the tension in the membrane, among other things\eta =0 at \rho =a.
(b) Separate variables and find the eigenfunctions.