(Solved): A particle in the harmonic oscillator potential is in the initial state: (x,0)=A[13m ...

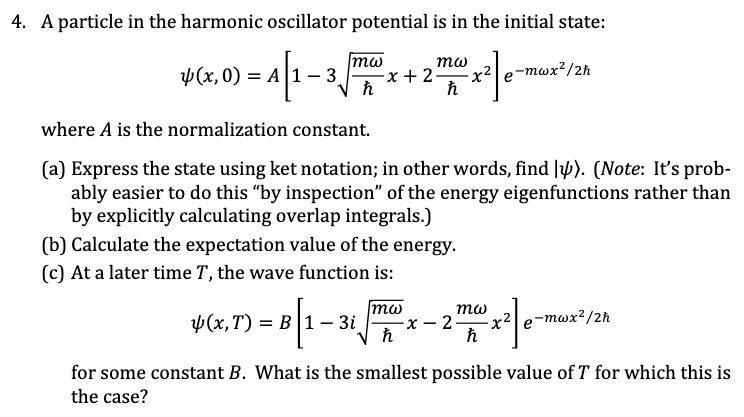
A particle in the harmonic oscillator potential is in the initial state: $?(x,0)=A\left[1?3\sqrt{\frac{m?}{?}}x+2\frac{m?}{?}{x}^2\right]e^{?m?x^2/2?}$ where $A$ is the normalization constant. (a) Express the state using ket notation; in other words, find $???$. (Note: It's probably easier to do this "by inspection" of the energy eigenfunctions rather than by explicitly calculating overlap integrals.) (b) Calculate the expectation value of the energy. (c) At a later time $T$, the wave function is: $?(x,T)=B\left[1?3i\sqrt{\frac{m?}{?}}x?2\frac{m?}{?}{x}^2\right]e^{?m?x^2/2?}$ for some constant $B$. What is the smallest possible value of $T$ for which this is the case?