(Solved): A probability density function in the exponential family is of the form: \( p(y ; \eta)= \) \( b(y ...

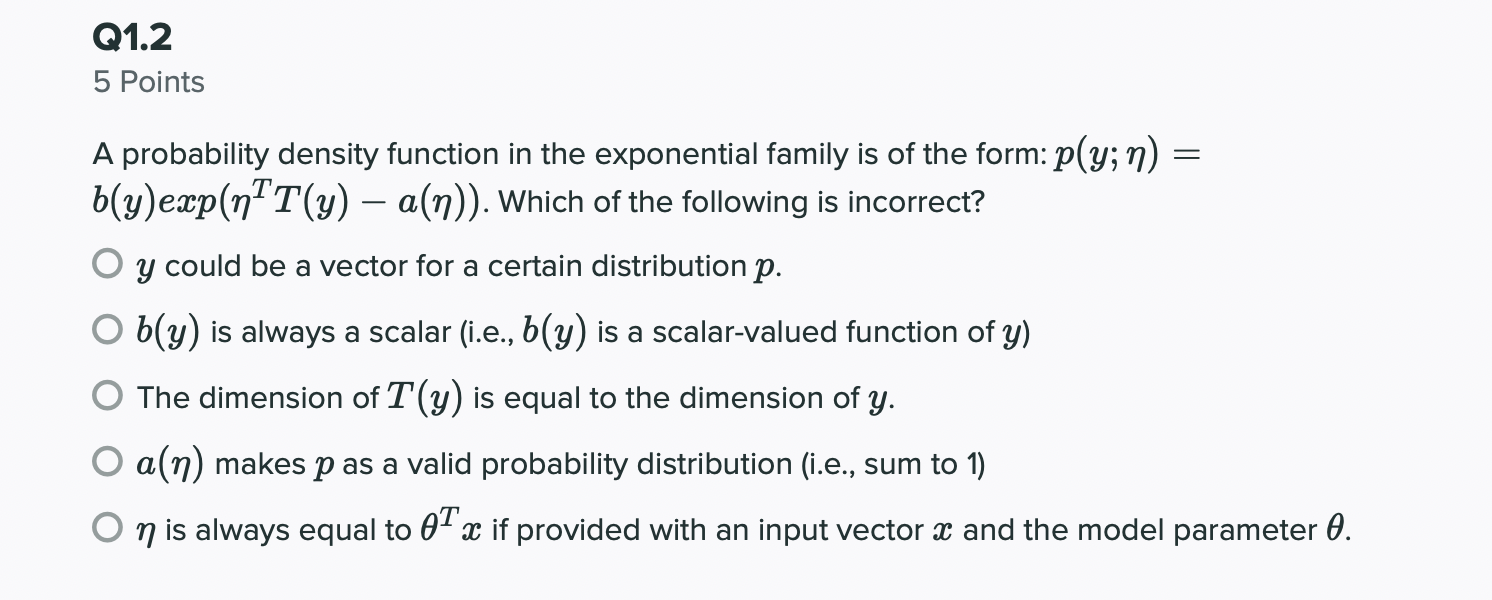
A probability density function in the exponential family is of the form: \( p(y ; \eta)= \) \( b(y) \exp \left(\eta^{T} T(y)-a(\eta)\right) \). Which of the following is incorrect? \( y \) could be a vector for a certain distribution \( p \). \( b(y) \) is always a scalar (i.e., \( b(y) \) is a scalar-valued function of \( y \) ) The dimension of \( T(y) \) is equal to the dimension of \( y \). \( a(\eta) \) makes \( p \) as a valid probability distribution (i.e., sum to 1) \( \eta \) is always equal to \( \theta^{T} x \) if provided with an input vector \( x \) and the model parameter \( \theta \). The univariate Gaussian density function is \( p\left(y ; \mu, \sigma^{2}\right)=\frac{1}{\sqrt{2 \pi} \sigma} \exp \left(\frac{(y-\mu)^{2}}{-2 \sigma^{2}}\right) \). Recall how we transformed this density into a form of exponential family in the lecture. Choose ALL correct descriptions. \( (\mu, \sigma) \) are called the standard parameter (i.e., parameters in the standard density format) The same density could be represented by multiple different forms. Natural parameter is the canonical parameter in the perspective of exponential family. The dimension of natural parameter could be different from the dimension of standard parameter for some distributions in the exponential family. If each outcome \( y \) is a result given an input \( x \), we can think model parameter \( \theta \) associates the input to the output (e.g., \( y=\theta^{T} x \) ). 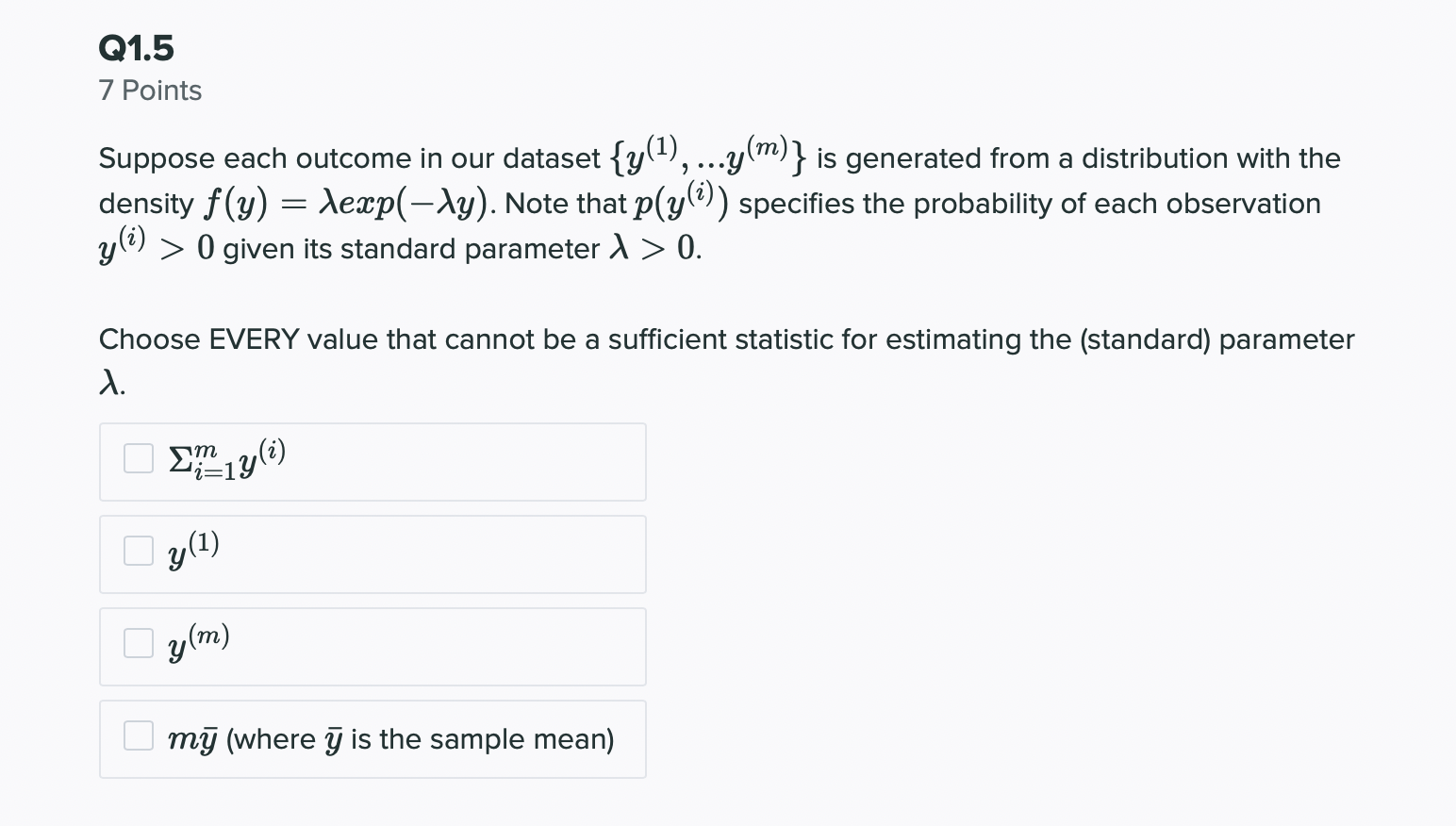Suppose each outcome in our dataset \( \left\{y^{(1)}, \ldots y^{(m)}\right\} \) is generated from a distribution with the density \( f(y)=\lambda \exp (-\lambda y) \). Note that \( p\left(y^{(i)}\right) \) specifies the probability of each observation \( y^{(i)}>0 \) given its standard parameter \( \lambda>0 \) Choose EVERY value that cannot be a sufficient statistic for estimating the (standard) parameter \( \lambda \) \[ \Sigma_{i=1}^{m} y^{(i)} \] \( y^{(m)} \) \( m \bar{y} \) (where \( \bar{y} \) is the sample mean)