A production function is given as below Y=K^( beta )L^(1- beta ) where Y is output, K is capital and L is effective worker. Suppose beta is 0.6 , growth rate of population (n) is 1%, depreciation rate of capital ( delta ) is 2% and growth rate of effective worker (g) is 4%. (a) If saving rate ( s ) is 30%, find steady states for capital per effec solutionspile.com

Question

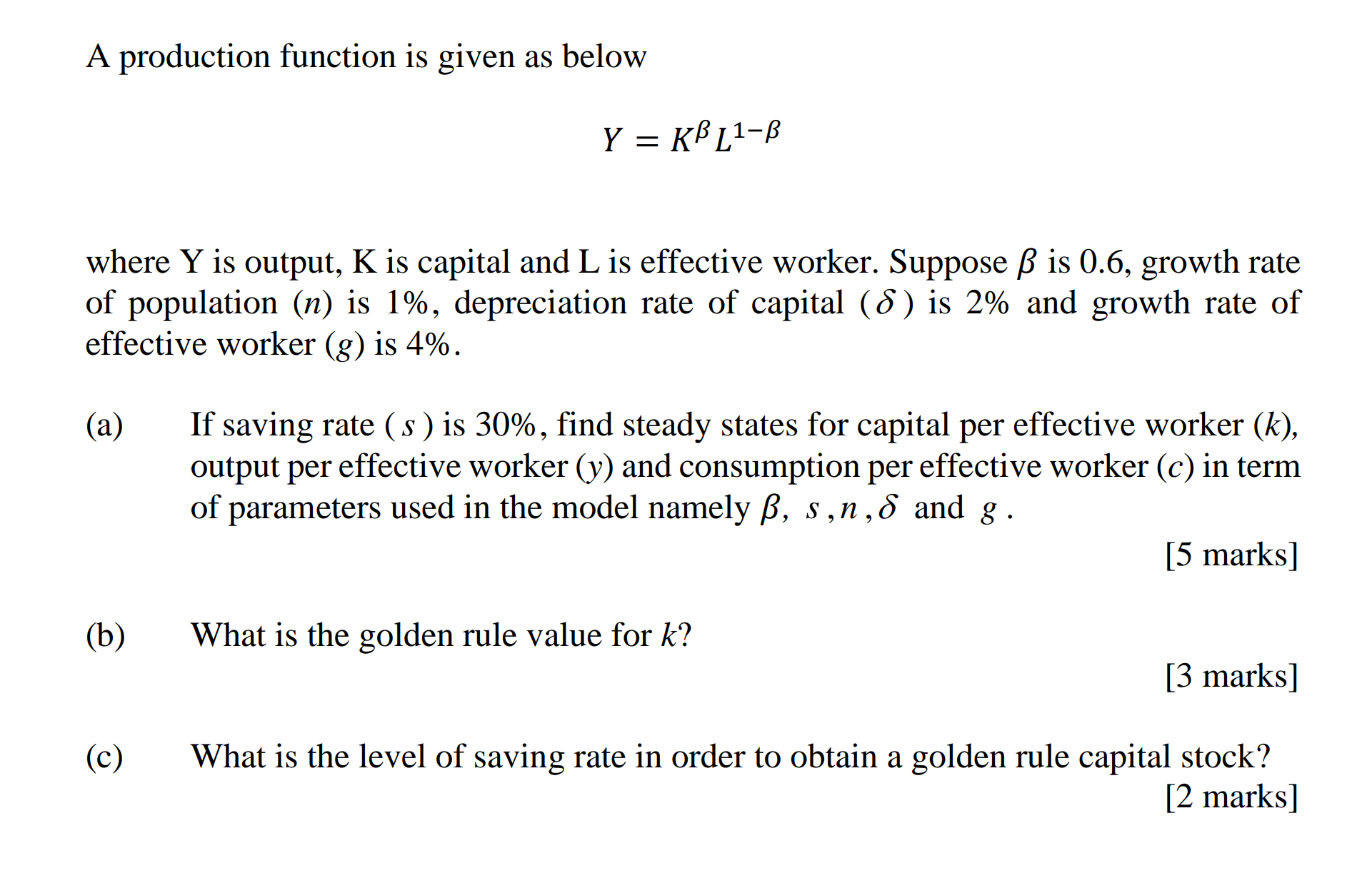
A production function is given as below

Y=K^(\beta )L^(1-\beta )

where Y is output, K is capital and L is effective worker. Suppose

\beta

is 0.6 , growth rate of population

(n)

is

1%

, depreciation rate of capital

(\delta )

is

2%

and growth rate of effective worker

(g)

is

4%

. (a) If saving rate (

s

) is 30%, find steady states for capital per effective worker

(k)

, output per effective worker

(y)

and consumption per effective worker

(c)

in term of parameters used in the model namely

\beta ,s,n,\delta

and

g

. [5 marks] (b) What is the golden rule value for

k

? [3 marks] (c) What is the level of saving rate in order to obtain a golden rule capital stock? [2 marks]

Accepted Answer

Expert Answer to - A production function is given as below Y=K^( beta )L^(1- beta ) where Y is output, K is capital and

Answer

Solution for - A production function is given as below Y=K^( beta )L^(1- beta ) where Y is output, K is capital and

Answer

This an additional answer to - A production function is given as below Y=K^( beta )L^(1- beta ) where Y is output, K is capital and

(Solved): A production function is given as below Y=K^(\beta )L^(1-\beta ) where Y is output, K is capital and ...