Home /
Expert Answers /
Advanced Math /
af-j-39-f-j-2-4f-j-1-3f-j-2-delta-delta-2-3-f-j-3-o-delta-3-f-j-39-pa826
(Solved): af_(j)^(')=(f_(j-2)-4f_(j-1)+3f_(j))/(2\Delta )+(\Delta ^(2))/(3)f_(j)^((3))+O(\Delta ^(3)) f_(j)^(' ...
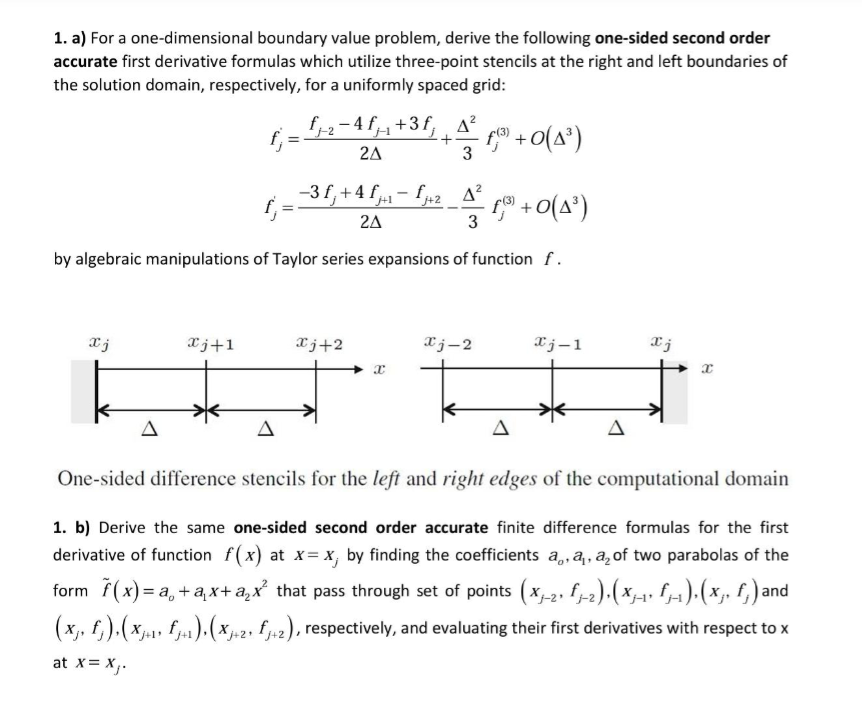
af_(j)^(')=(f_(j-2)-4f_(j-1)+3f_(j))/(2\Delta )+(\Delta ^(2))/(3)f_(j)^((3))+O(\Delta ^(3))
f_(j)^(')=(-3f_(j)+4f_(j+1)-f_(j+2))/(2\Delta )-(\Delta ^(2))/(3)f_(j)^((3))+O(\Delta ^(3))
by algebraic manipulations of Taylor series expansions of function f.
One-sided difference stencils for the left and right edges of the computational domain
bf(x) at x=x_(j) by finding the coefficients a_(o),a_(1),a_(2) of two parabolas of the
form tilde(f)(x)=a_(o)+a_(1)x+a_(2)x^(2) that pass through set of points (x_(j-2),f_(j-2)),(x_(j-1),f_(j-1)),(x_(j),f_(j)) and
(x_(j),f_(j)),(x_(j+1),f_(j+1)),(x_(j+2),f_(j+2)), respectively, and evaluating their first derivatives with respect to x
at x=x_(j).