Home /
Expert Answers /
Advanced Math /
b-let-f-r-denote-the-set-of-all-functions-from-r-into-r-i-show-that-f-r-is-a-vector-space-over-pa636
(Solved): (b) Let F(R) denote the set of all functions from R into R. i. Show that F(R) is a vector space over ...
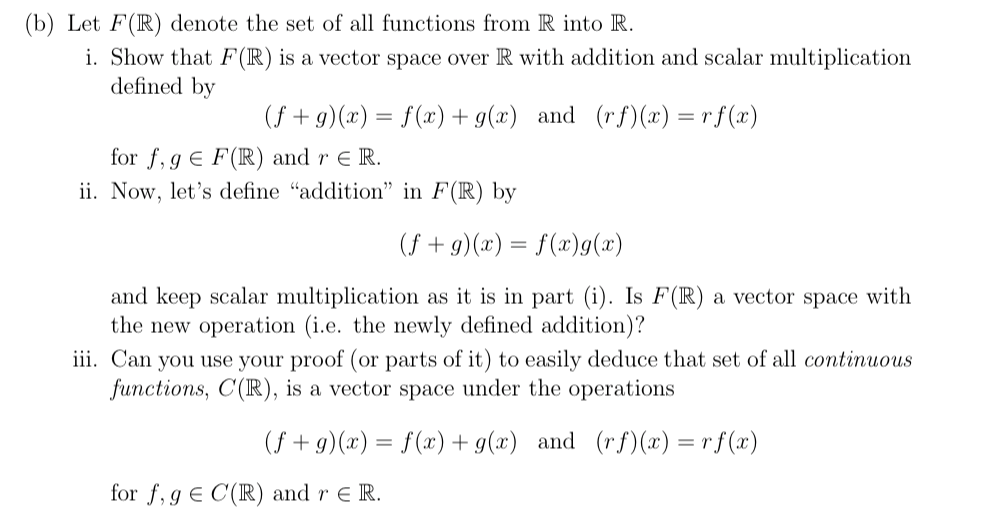
(b) Let F(R) denote the set of all functions from R into R.
i. Show that F(R) is a vector space over R with addition and scalar multiplication
defined by
(f+g)(x)=f(x)+g(x), and ,(rf)(x)=rf(x)
for f,ginF(R) and rinR.
ii. Now, let's define "addition" in F(R) by
(f+g)(x)=f(x)g(x)
and keep scalar multiplication as it is in part (i). Is F(R) a vector space with
the new operation (i.e. the newly defined addition)?
iii. Can you use your proof (or parts of it) to easily deduce that set of all continuous
functions, C(R), is a vector space under the operations
(f+g)(x)=f(x)+g(x) and (rf)(x)=rf(x)
for f,ginC(R) and rinR.