Home /
Expert Answers /
Mechanical Engineering /
consider-a-mechanical-system-consisting-of-a-spring-with-stiffness-k-2500-n-m-a-viscous-damping-pa598
(Solved): Consider a mechanical system consisting of a spring with stiffness K=2500(N)/(m), a viscous damping ...
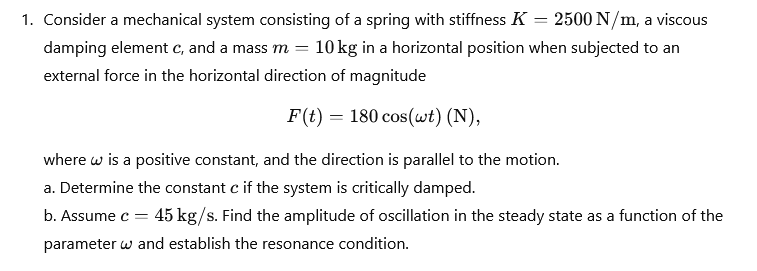
Consider a mechanical system consisting of a spring with stiffness K=2500(N)/(m), a viscous
damping element c, and a mass m=10kg in a horizontal position when subjected to an
external force in the horizontal direction of magnitude
F(t)=180cos(\omega t) (N)
where \omega is a positive constant, and the direction is parallel to the motion.
a. Determine the constant c if the system is critically damped.
b. Assume c=45k(g)/(s). Find the amplitude of oscillation in the steady state as a function of the
parameter \omega and establish the resonance condition.