(Solved): Consider two vectors \( \vec{A} \) and \( \vec{B} \) and their resultant \( \vec{A}+\vec{B} \). Th ...

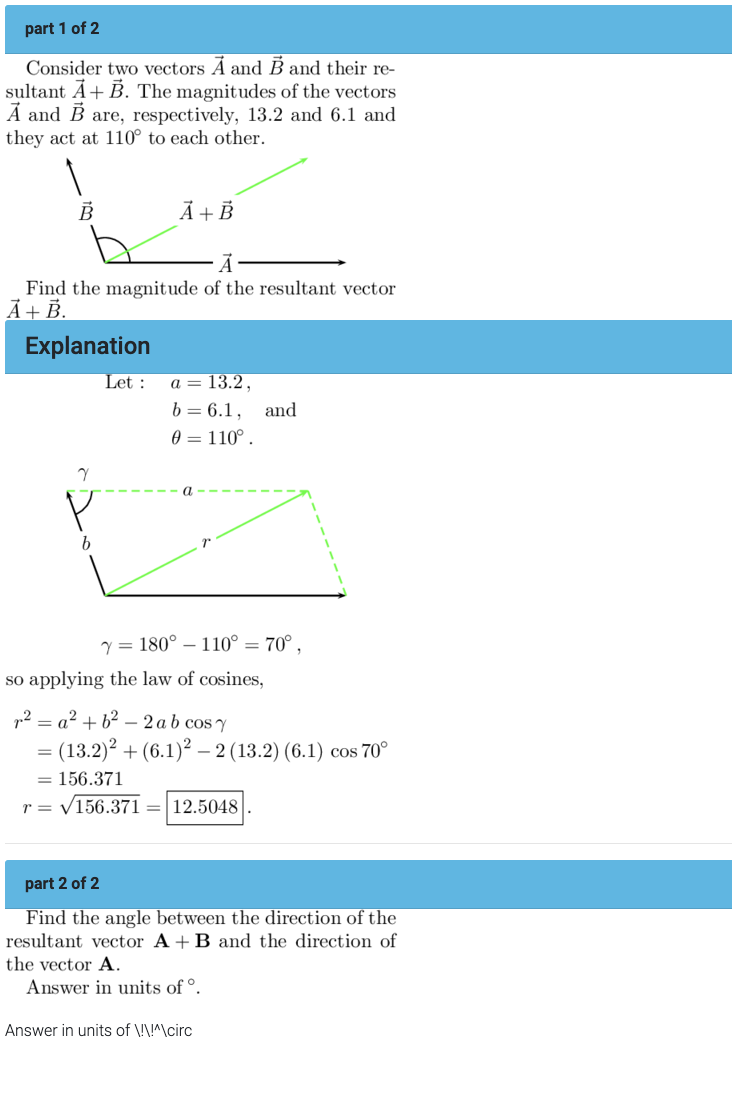
Consider two vectors \( \vec{A} \) and \( \vec{B} \) and their resultant \( \vec{A}+\vec{B} \). The magnitudes of the vectors \( \vec{A} \) and \( \vec{B} \) are, respectively, \( 13.2 \) and \( 6.1 \) and they act at \( 110^{\circ} \) to each other. Find the magnitude of the resultant vector \( \vec{A}+\vec{B} \) Explanation Let : \( a=13.2 \), \( b=6.1, \quad \) and \( \theta=110^{\circ} \) \[ \gamma=180^{\circ}-110^{\circ}=70^{\circ}, \] so applying the law of cosines, \[ \begin{aligned} r^{2} &=a^{2}+b^{2}-2 a b \cos \gamma \\ &=(13.2)^{2}+(6.1)^{2}-2(13.2)(6.1) \cos 70^{\circ} \\ &=156.371 \\ r &=\sqrt{156.371}= \end{aligned} \] part 2 of 2 Find the angle between the direction of the resultant vector \( \mathbf{A}+\mathbf{B} \) and the direction of the vector \( \mathbf{A} \). Answer in units of \( { }^{\circ} \).