(Solved): \( \delta(t) \) is Dirac delta, \( * \) is convolution, \( \quad \star \) is correlation, \( \qua ...

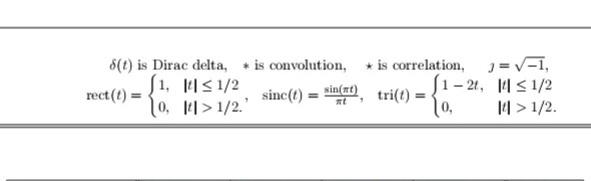
\( \delta(t) \) is Dirac delta, \( * \) is convolution, \( \quad \star \) is correlation, \( \quad J=\sqrt{-1} \), \( \operatorname{rect}(t)=\left\{\begin{array}{ll}1, & |t| \leq 1 / 2 \\ 0, & |t|>1 / 2 .\end{array} \quad \operatorname{sinc}(t)=\frac{\sin (\pi t)}{\pi t}, \quad \operatorname{tri}(t)=\left\{\begin{array}{ll}1-2 t, & |t| \leq 1 / 2 \\ 0, & |t|>1 / 2 .\end{array}\right.\right. \) 1. Calculate the expressions (a) \[ f_{1}(t)=\left(t^{2}-1\right) \delta(t-2)+\frac{e^{-t}-1}{t} \delta(t-1)+\frac{e^{-t}-1}{t} * \delta(t-1)+\delta(t+1) \delta(t-1) . \] (b) \[ f_{2}(t)=x(a-t) \delta(b-t)+x(a-t) * \delta(b-t)+x(t+b) * \delta(t+b)+x(t+a) \delta(t+(2)) \] 2. Calculate the convolutions (a) \[ f_{3}(t)=(t u(t-b)) * u(t+a) . \] (b) \[ f_{4}(t)=\left(t^{2} \delta(r-t)\right) * \delta(t-r) . \] 3. Calculate (a) \[ f_{5}(t)=\operatorname{sinc}(t) \cdot \sum_{k=-\infty}^{\infty} \delta(t-k) . \] (b) \[ f_{6}(t)=\int_{-2}^{2}(t+2)^{3} \delta(t-1) d t+\int_{-\infty}^{\infty}(t+2)^{3} \delta(t-1) d t+\int_{2}^{\infty}(t+2)^{3} \delta(t-1) d t .(6) \] 4. Calculate the even and the odd part of the functuion \[ f_{7}(t)=\frac{1-t}{1+t} . \]