Home /
Expert Answers /
Mechanical Engineering /
find-the-flow-rate-q-between-two-reservoirs-connected-by-two-pipes-in-series-with-different-diame-pa728
(Solved): :Find the flow rate, Q, between two reservoirs connected by two pipes in series with different diame ...
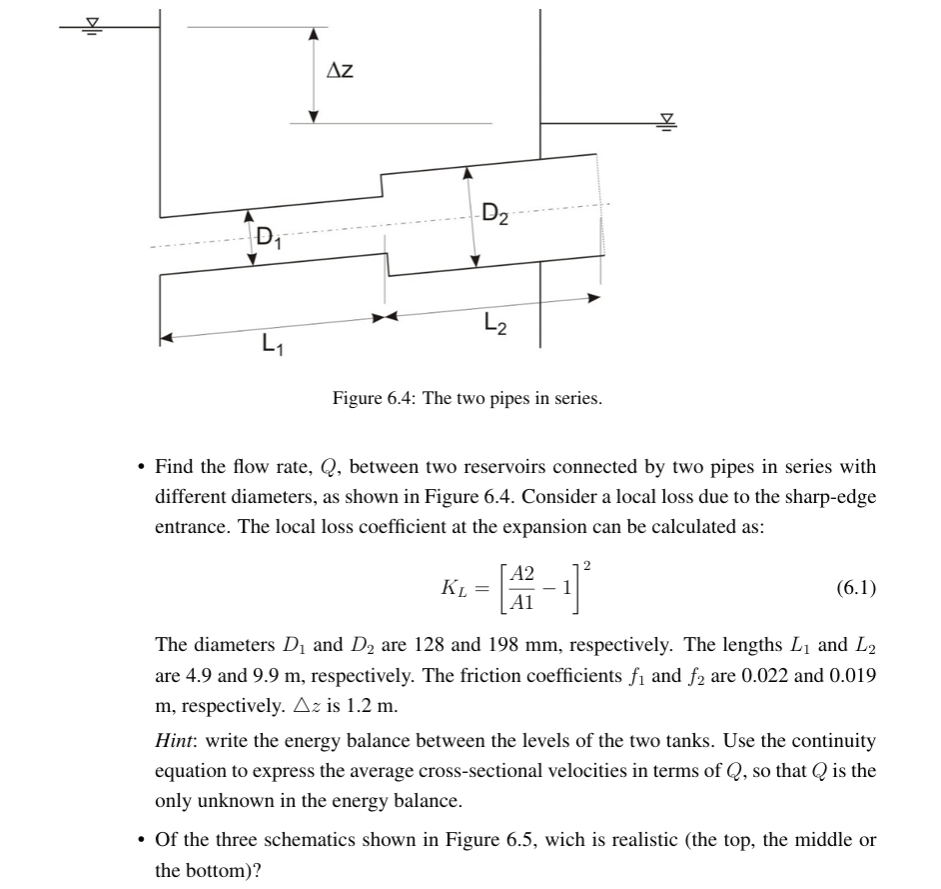
:Find the flow rate, Q, between two reservoirs connected by two pipes in series with different diameters, as shown in Figure 6.4. Consider a local loss due to the sharp-edge entrance. The local loss coefficient at the expansion can be calculated as: Figure 6.4: The two pipes in series.
Find the flow rate, Q, between two reservoirs connected by two pipes in series with different diameters, as shown in Figure 6.4. Consider a local loss due to the sharp-edge entrance. The local loss coefficient at the expansion can be calculated as:
K_(L)=[(A2)/(A1)-1]^(2)
The diameters D_(1) and D_(2) are 128 and 198 mm , respectively. The lengths L_(1) and L_(2) are 4.9 and 9.9 m , respectively. The friction coefficients f_(1) and f_(2) are 0.022 and 0.019 m , respectively. \Delta z is 1.2 m .
Hint: write the energy balance between the levels of the two tanks. Use the continuity equation to express the average cross-sectional velocities in terms of Q, so that Q is the only unknown in the energy balance.
Of the three schematics shown in Figure 6.5, wich is realistic (the top, the middle or the bottom)?