Home /
Expert Answers /
Calculus /
find-the-two-one-sided-limits-as-x-gt-3-of-the-floor-function-f-x-x-vdots-if-5-l-pa232
(Solved): Find the two one-sided limits as x->-3 of the floor function f(x)=|_(_())x_(_())|={(vdots if -5&l ...
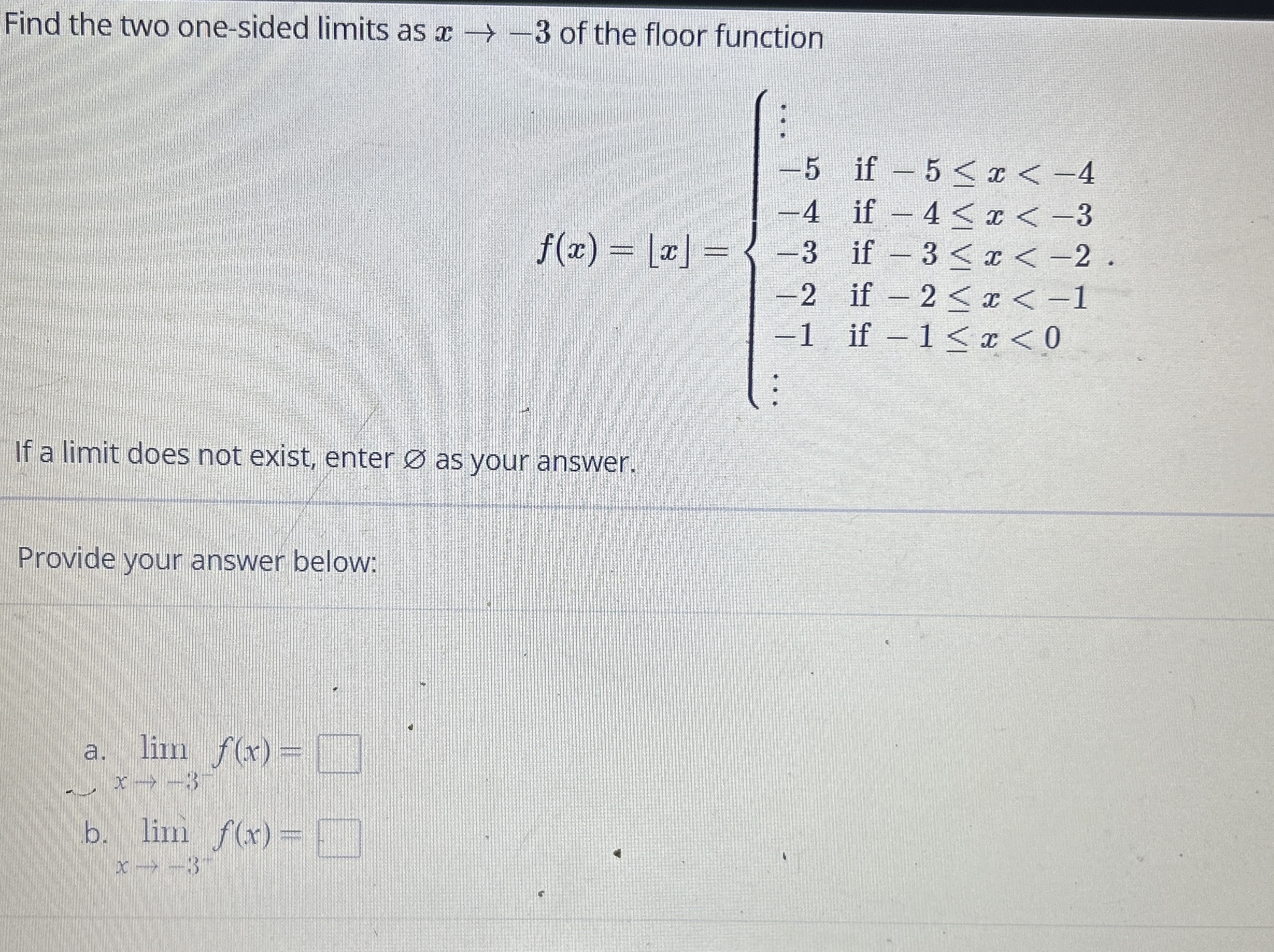
Find the two one-sided limits as
x->-3of the floor function
f(x)=|_(_())x_(_())|={(vdots if -5<=x<-4),(-5 if -4<=x<-3),(-4 if -3<=x<-2),(-3 if -3<=x<-1),(-2 if -2<=x<),(-1 if -1<=x<0),(vdots,):}If a limit does not exist, enter
(O)/()as your answer. Provide your answer below: a.
\lim_()f(x)=
\lim_(x->-3^(-))f(x)=?