(Solved): Given a pendulum of length L and a point mass M with input u(t) as the force applied tangential to ...

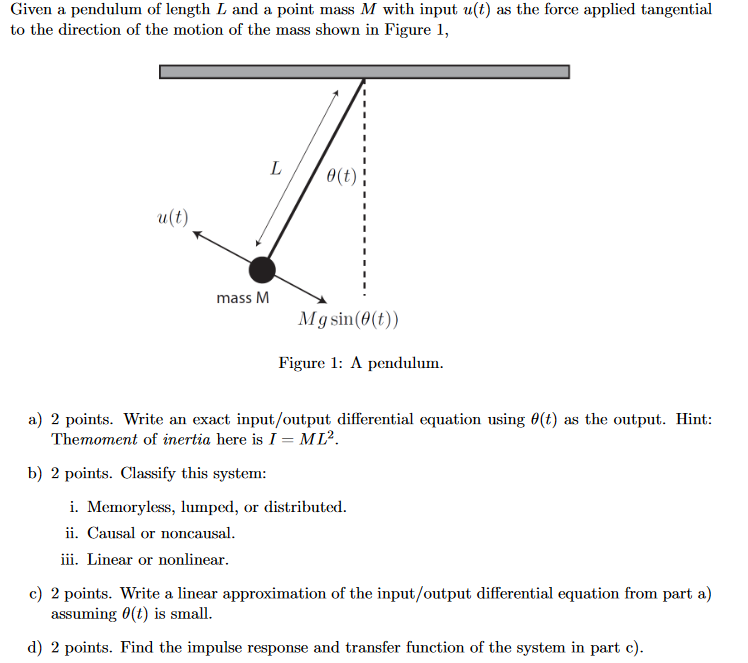
Given a pendulum of length

and a point mass

with input

as the force applied tangential to the direction of the motion of the mass shown in Figure 1, Figure 1: A pendulum. a) 2 points. Write an exact input/output differential equation using

as the output. Hint: Themoment of inertia here is

. b) 2 points. Classify this system: i. Memoryless, lumped, or distributed. ii. Causal or noncausal. iii. Linear or nonlinear. c) 2 points. Write a linear approximation of the input/output differential equation from part a) assuming

is small. d) 2 points. Find the impulse response and transfer function of the system in part c).