(Solved): Historically, the SAT score has unknown distribution with a mean of 1484 points and a standard devi ...

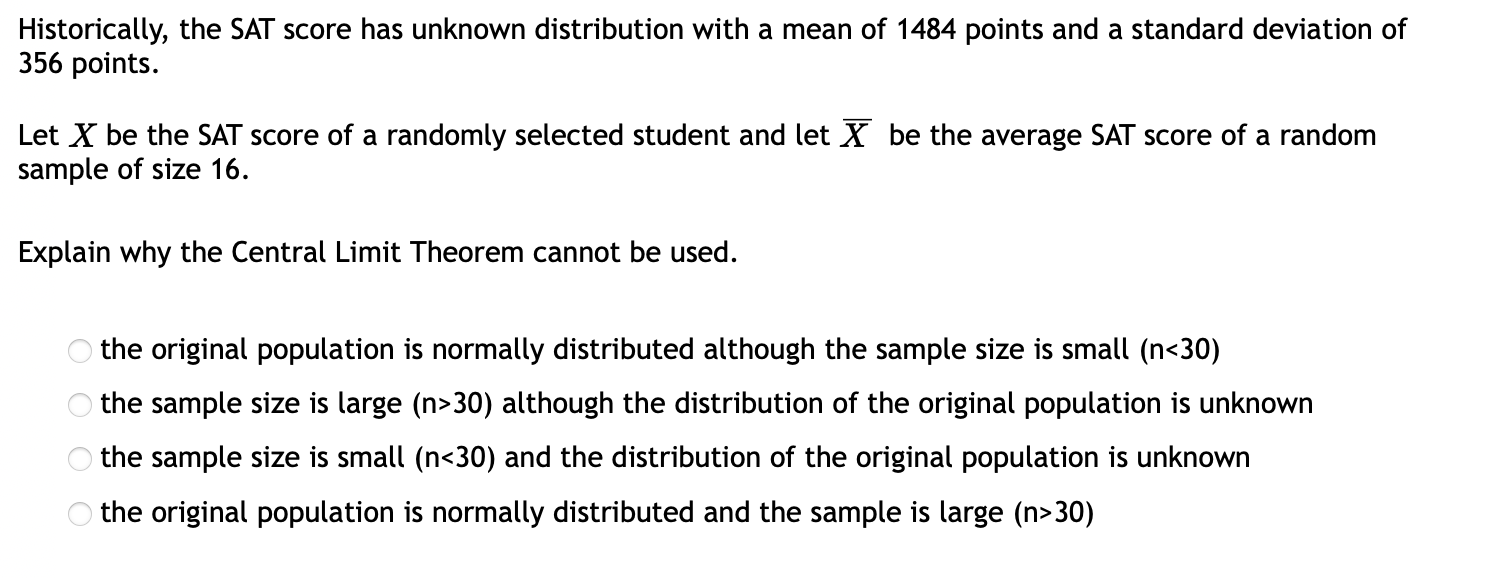
Historically, the SAT score has unknown distribution with a mean of 1484 points and a standard deviation of 356 points. Let \( X \) be the SAT score of a randomly selected student and let \( \bar{X} \) be the average SAT score of a random sample of size 16. Explain why the Central Limit Theorem cannot be used. the original population is normally distributed although the sample size is small ( \( \mathrm{n}<30 \) ) the sample size is large \( (\mathrm{n}>30) \) although the distribution of the original population is unknown the sample size is small \( (\mathrm{n}<30) \) and the distribution of the original population is unknown the original population is normally distributed and the sample is large ( \( n>30 \) )