Home /
Expert Answers /
Calculus /
in-this-exercise-we-characterize-the-area-of-the-sphere-of-radius-r-in-n-dimensions-denoted-s-n-pa977
(Solved): In this exercise, we characterize the area of the sphere of radius R in n dimensions, denoted S_(n)( ...
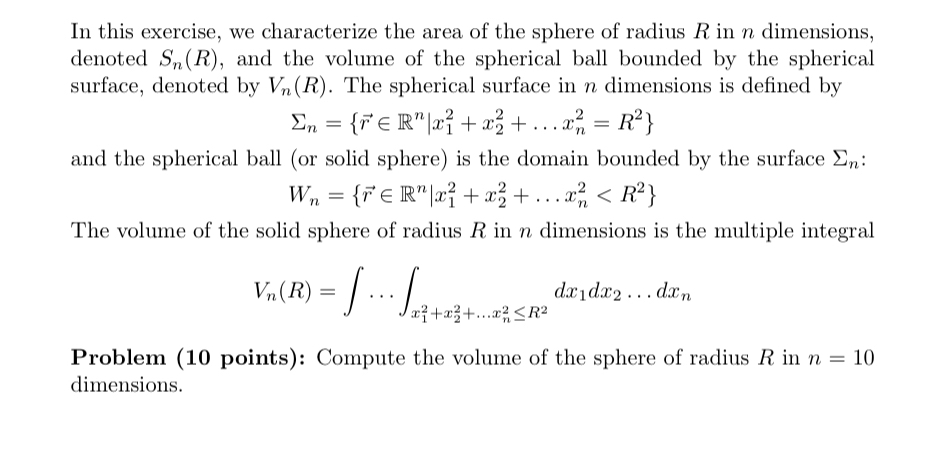
In this exercise, we characterize the area of the sphere of radius
Rin
ndimensions, denoted
S_(n)(R), and the volume of the spherical ball bounded by the spherical surface, denoted by
V_(n)(R). The spherical surface in
ndimensions is defined by
\Sigma _(n)={(vec(r))inR^(n)|x_(1)^(2)+x_(2)^(2)+dotsx_(n)^(2)=R^(2)}and the spherical ball (or solid sphere) is the domain bounded by the surface
\Sigma _(n):
RnV_(n)(R)=\int dots\int_(x_(1)^(2)+x_(2)^(2)+dotsx_(n)^(2)<=R^(2)) dx_(1)dx_(2)dotsdx_(n)Rn=10