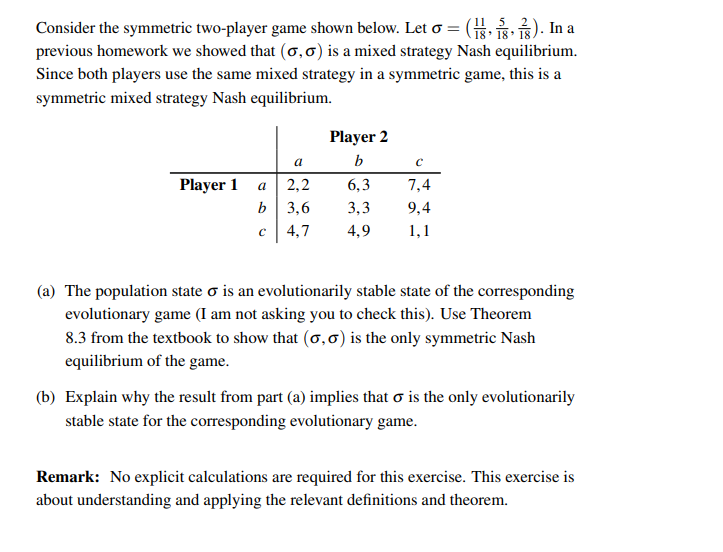
(Solved): L8 GAME THEORY: Consider the symmetric two-player game shown below. Let \sigma =((11)/(18),(5)/(18) ...
L8 GAME THEORY: Consider the symmetric two-player game shown below. Let
\sigma =((11)/(18),(5)/(18),(2)/(18)). In a previous homework we showed that
(\sigma ,\sigma )is a mixed strategy Nash equilibrium. Since both players use the same mixed strategy in a symmetric game, this is a symmetric mixed strategy Nash equilibrium. (a) The population state
\sigma is an evolutionarily stable state of the corresponding evolutionary game (I am not asking you to check this). Use Theorem 8.3 from the textbook to show that
(\sigma ,\sigma )is the only symmetric Nash equilibrium of the game. (b) Explain why the result from part (a) implies that
\sigma is the only evolutionarily stable state for the corresponding evolutionary game. Remark: No explicit calculations are required for this exercise. This exercise is about understanding and applying the relevant definitions and theorem.