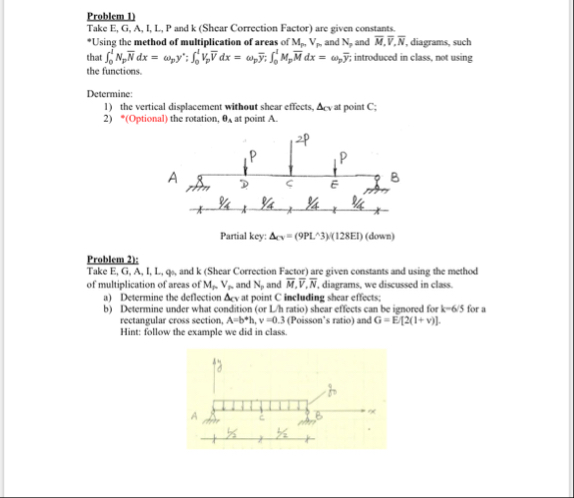
(Solved): Problem.1 Take E, G, A, I, L, P and k (Shear Correction Factor) are given constants. ^(**) Using the ...
Problem.1 Take E, G, A, I, L, P and k (Shear Correction Factor) are given constants.
^(**)Using the method of multiplication of areas of
M_(p),V_(p)and
N_(p)and
/bar (M)(,)/(b)ar (V)(,)/(b)ar (N), diagrams, such that
\int_0^l (N_(p))/(b)ar (N)dx=\omega _(p)y^(**);\int_0^l (V_(p))/(b)ar (V)dx=(\omega _(p))/(b)ar (y);\int_0^l (M_(p))/(b)ar (M)dx=(\omega _(p))/(b)ar (y); introduced in class, not using the functions. Determine: the vertical displacement without shear effects,
\Delta _(Cv)at point C ; *(Optional) the rotation,
\theta _(A)at point A . Partial key:
\Delta cv=(9PL^(^())3)(128E1)(down) Problem 2): Take E, G, A, I, L, qs, and k (Shear Correction Factor) are given constants and using the method of multiplication of areas of
M_(p)V_(p)and
N_(p)and
/bar (M)(,)/(b)ar (V)(,)/(b)ar (N), diagrams, we discussed in class. a) Determine the deflection
\Delta _(cv)at point C including shear effects; b) Determine under what condition (or L/h ratio) shear effects can be ignored for
k=(6)/(5)for a rectangular cross section,
A=b^( )h,v=0.3(Poisson's ratio) and
G=E[2(1 v)]. Hint: follow the example we did in class.