(Solved): Question 1 - Closed-loop transfer functions The figure above shows a unity-feedback closed-loop co ...

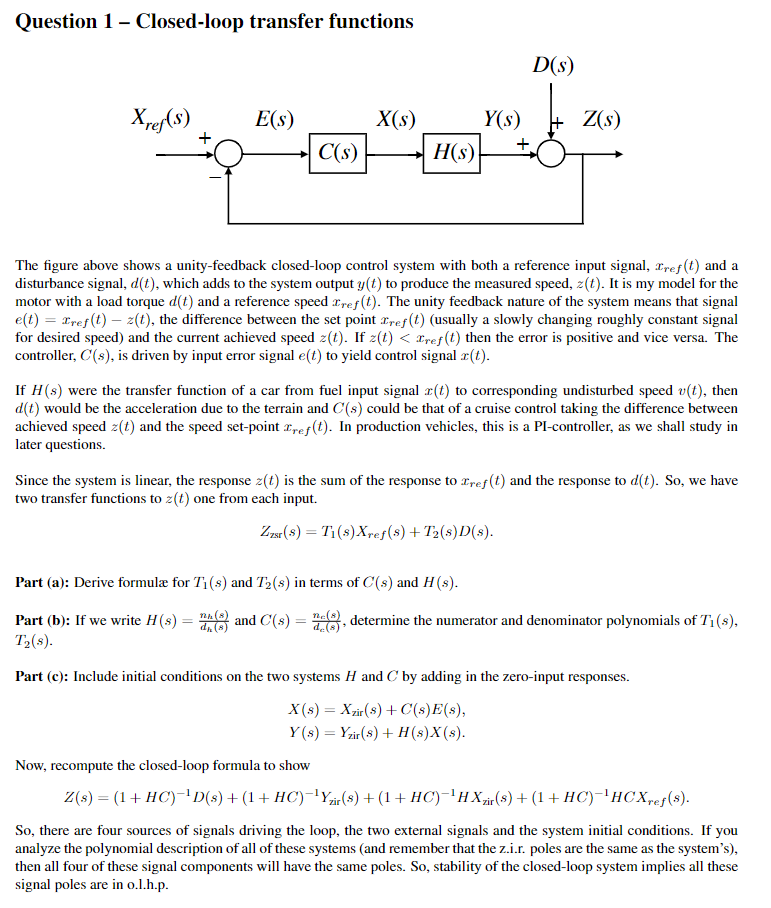
Question 1 - Closed-loop transfer functions The figure above shows a unity-feedback closed-loop control system with both a reference input signal, $$x_{ref}(t)$$ and a disturbance signal, $$d(t)$$, which adds to the system output $$y(t)$$ to produce the measured speed, $$z(t)$$. It is my model for the motor with a load torque $$d(t)$$ and a reference speed $$x_{ref}(t)$$. The unity feedback nature of the system means that signal $$e(t)=x_{ref}(t)?z(t)$$, the difference between the set point $$x_{ref}(t)$$ (usually a slowly changing roughly constant signal for desired speed) and the current achieved speed $$z(t)$$. If $$z(t)<x_{ref}(t)$$ then the error is positive and vice versa. The controller, $$C(s)$$, is driven by input error signal $$e(t)$$ to yield control signal $$x(t)$$. If $$H(s)$$ were the transfer function of a car from fuel input signal $$x(t)$$ to corresponding undisturbed speed $$v(t)$$, then $$d(t)$$ would be the acceleration due to the terrain and $$C(s)$$ could be that of a cruise control taking the difference between achieved speed $$z(t)$$ and the speed set-point $$x_{ref}(t)$$. In production vehicles, this is a PI-controller, as we shall study in later questions. Since the system is linear, the response $$z(t)$$ is the sum of the response to $$x_{ref}(t)$$ and the response to $$d(t)$$. So, we have two transfer functions to $$z(t)$$ one from each input. $$Z_{\mathrm{zsr}}(s)=T_1(s)X_{\text{ref }}(s)+T_2(s)D(s).$$ Part (a): Derive formulæ for $$T_1(s)$$ and $$T_2(s)$$ in terms of $$C(s)$$ and $$H(s)$$. Part (b): If we write $$H(s)=\frac{n_h(s)}{d_h(s)}$$ and $$C(s)=\frac{n_c(s)}{d_c(s)}$$, determine the numerator and denominator polynomials of $$T_1(s)$$, $$T_2(s)$$ Part (c): Include initial conditions on the two systems $$H$$ and $$C$$ by adding in the zero-input responses. $$\begin{array}{l}X(s)=X_{\mathrm{zir}}(s)+C(s)E(s),\\ Y(s)=Y_{\mathrm{zir}}(s)+H(s)X(s).\end{array}$$ Now, recompute the closed-loop formula to show $$Z(s)=(1+HC{)}^{?1}D(s)+(1+HC{)}^{?1}{Y}_{\text{zir }}(s)+(1+HC{)}^{?1}H{X}_{\text{zir }}(s)+(1+HC{)}^{?1}HC{X}_{ref}(s).$$ So, there are four sources of signals driving the loop, the two external signals and the system initial conditions. If you analyze the polynomial description of all of these systems (and remember that the z.i.r. poles are the same as the system's), then all four of these signal components will have the same poles. So, stability of the closed-loop system implies all these signal poles are in o.l.h.p.