Home /
Expert Answers /
Mechanical Engineering /
question-1-in-fluid-mechanics-the-material-derivative-is-used-to-describe-the-rate-of-change-of-a-p-pa478
(Solved): QUESTION 1 In fluid mechanics, the material derivative is used to describe the rate of change of a p ...
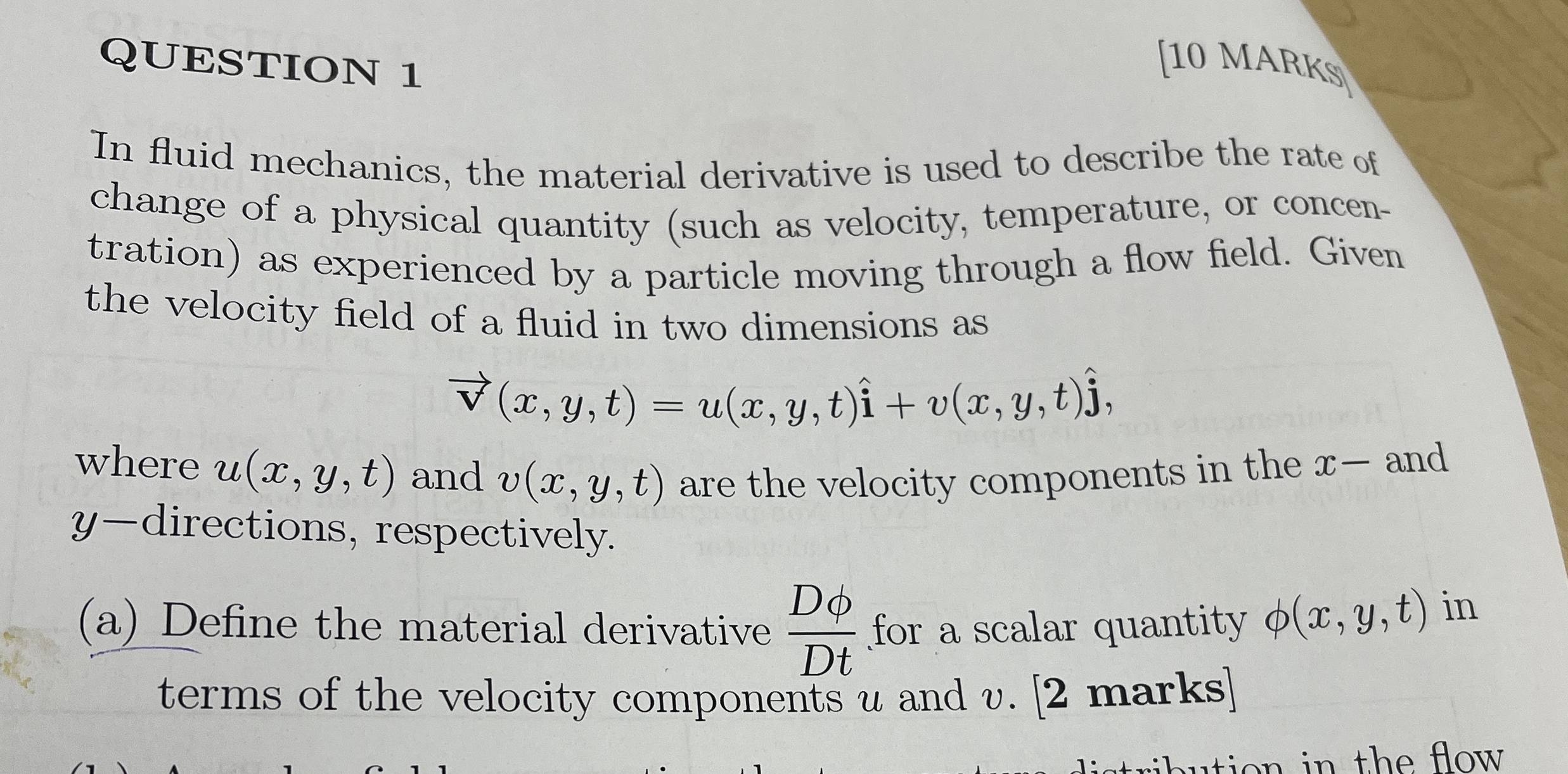
QUESTION 1 In fluid mechanics, the material derivative is used to describe the rate of change of a physical quantity (such as velocity, temperature, or concen- tration) as experienced by a particle moving through a flow field. Given the velocity field of a fluid in two dimensions as
vec(V)(x,y,t)=u(x,y,t)hat(i)+v(x,y,t)hat(j)where
u(x,y,t)and
v(x,y,t)are the velocity components in the
x- and
y-directions, respectively. (a) Define the material derivative
(D\phi )/(Dt)for a scalar quantity
\phi (x,y,t)in terms of the velocity components
uand
v. [2 marks]